What is NaysCUBE?

NaysCUBE is an unsteady fully three-dimensional solver for river flow and bed morphodynamics written by Professor Ichiro Kimura of Toyama University. The approach solves the basic equations of three-dimensional flow incorporate the nonhydrostatic water pressure associated with strong vertical accelerations and velocities. The user can choose from a variety of turbulence closures including a non-isotropic higher-order k-ε treatment. This solver can simulate fully three-dimensional flow phenomena, such as the secondary current of the first second kind, the horseshoe vortex around river structures, as well as the associated bed morphodynamic features. This solver covers a wider range of phenomena compared with 2D models, although it requires one-order (or more) larger CPU time. Therefore, this solver is suitable to be applied on a limited river reach for detailed analysis.

The following figure shows an example of simulation of secondary flow of the first kind at a river bend.

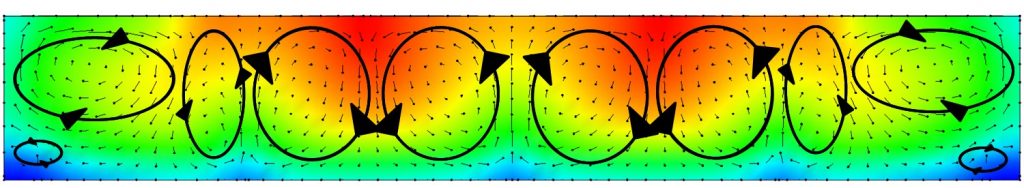
The following figure is an example of the simulation of the secondary current of the second kind in a rectangular straight open channel flow.

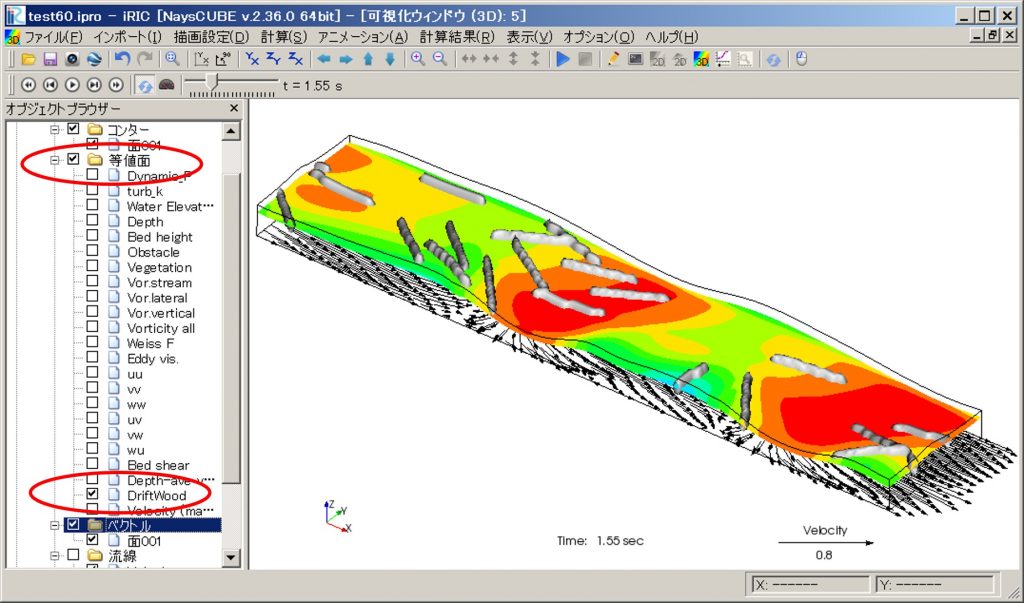
NaysCUBE ver.3 also includes a new module for simulating driftwood or arbitrarily shaped objects transport as shown in the figure below.

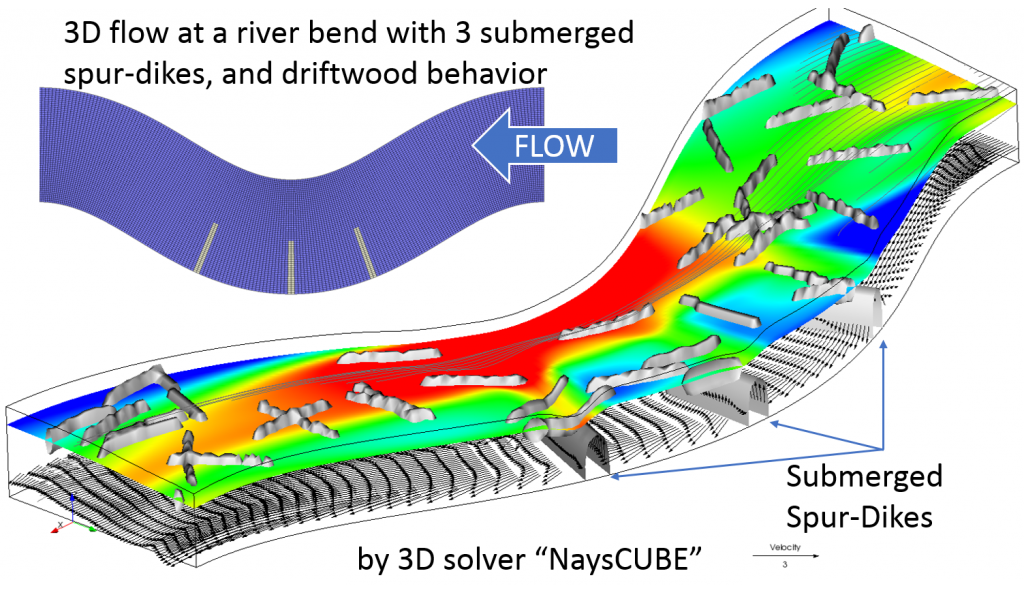
This solver employs a generalized curvilinear coordinate system which is transforms the complicated river topography to a group of cubes. The name of the solver comes from this transformation process.

img

Try “NaysCUBE Example (Real River Version)” and enjoy the interesting world of 3D simulations for rivers!